Obtaining the momentum distribution from $g_1(r)$

The asymptotic behaviour of the one body density matrix of the Lieb gas is

$$\rho(x) = \frac{C}{x^\alpha}, \qquad x \gg 1$$ (11.1)

In order to calculate the momentum distribution one has to calculate the Fourier transform of it

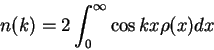$$n(k) = 2 \int_0^\infty \cos kx \rho(x) dx$$ (11.2)

This integral can be calculated numerically up to some cut-off distance $L$. Let us suppose, that at distances larger than $L$ the asymptotic behavior is valid (B.1). Than one can calculate the ``tail'' integral analytically^11.1by a substitution $t = e^{i\pi/2}kx$

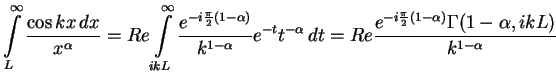$$\int\limits_L^\infty \frac{\cos kx\,dx}{x^\alpha} = Re \int\limit... ...dt = Re \frac{e^{-i\frac{\pi}{2}(1-\alpha)}\Gamma(1-\alpha, ikL)}{k^{1-\alpha}}$$ (11.3)

Here the incomplete Gamma function is defined as

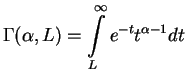$$\Gamma(\alpha, L) = \int\limits_L^\infty e^{-t} t^{\alpha-1}dt$$ (11.4)

If the if set $L=0$ then the integral can be simplified^11.2

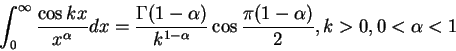$$\int_0^\infty \frac{\cos kx}{x^{\alpha}} dx = \frac{\Gamma(1... ...)}{k^{1-\alpha}} \cos \frac{\pi(1-\alpha)}{2}, k>0, 0<\alpha<1$$ (11.5)

Let us derive an expansion of the incomplete Gamma function in terms of $1/(kL)$. For us it is convenient to use following definition of the function

$$f(k,L,\alpha) = \int\limits_L^\infty \frac{\cos\,kx}{x^\alpha}\,dx$$ (11.6)

Integrating it by parts two times we obtain^11.3

$$f(k,L,\alpha) = \frac{1}{kL^\alpha} \left(-\sin\,kL+\frac{\alpha}... ...c{\alpha(\alpha+1)}{k^2} \int\limits_L^\infty \frac{\cos\,kx}{x^{\alpha+2}}\,dx$$ (11.7)

Here the last term has the same form as (B.6). And can be expanded in a similar way. Continuation of this expansion leads to formula

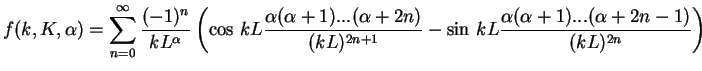$$f(k,K,\alpha) = \sum\limits_{n=0}^\infty \frac{(-1)^n}{kL^\alpha}... ...kL)^{2n+1}} -\sin\,kL\frac{\alpha(\alpha+1)...(\alpha+2n-1)}{(kL)^{2n}} \right)$$ (11.8)

Another way to present it is

$$f(k,K,\alpha) = \frac{1}{kL^\alpha} \sum\limits_{n=0}^\infty \frac{\mathop{\rm Im} i^ne^{-ikL}}{(kL)^n} \frac{(\alpha+n-1)!}{(\alpha-1)!}$$ (11.9)

Let us write explicitly

$$\mathop{\rm Im} i^ne^{-ikL} = (-1)^{\mathop{\rm mod}(n+1,2)+1} f_n(kL)$$ (11.10)

where $\mathop{\rm mod}$ operation is integer division and the function $f_n$ is defined as

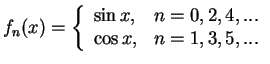$$f_n(x) = \left\{ \begin{array}{lr} \sin x,&n=0,2,4,...\\ \cos x,&n=1,3,5,... \end{array}\right.$$ (11.11)

Looking at the structure of the expansion (B.9) one finds out that the sum converges only is $kL>1$. The factorial dependence of the numerator on the order of the term $n$ leads to divergence of the entire sum. Let us find order of the term $n_{cr}$ when the summation procedure should be stopped. The condition is

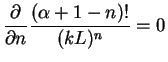$$\frac{\partial}{\partial n} \frac{(\alpha+1-n)!}{(kL)^n} = 0$$ (11.12)

In order to proceed further we will take use of the Stirling formula

$$n! = \sqrt{2\pi n} n^n e^{-n}$$ (11.13)

Simple calculation gives

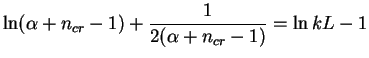$$\ln(\alpha+n_{cr}-1)+\frac{1}{2(\alpha+n_{cr}-1)} = \ln kL -1$$ (11.14)

Now we assume that $n$ is much larger than one, so we can neglect the second term. Finally we obtain

$$n_{cr} = kL/e$$ (11.15)

Another approach for the calculation of the integral is by modifying the integration contour. First of all, let us expand the cosine into sum of complex exponents

$$\int_L^\infty \frac{\cos x\,dx}{x^\alpha} = \frac{1}{2}\int_L^\in... ...c{e^{ix}\,dx}{x^\alpha} + \frac{1}{2}\int_L^\infty \frac{e^{-ix}\,dx}{x^\alpha}$$ (11.16)

Let us calculate the first integral in this sum.

$$I_1 = \int\limits_L^\infty \frac{e^{ix}\,dx}{x^\alpha} = \int\limits_0^\infty \frac{e^{-y} e^{iL}\,idy}{(iy+L)^\alpha},$$ (11.17)

where we introduced notation $x=iy+L$, which is a complex variable $x = \sqrt{y^2+L^2} e^{i\mathop{\rm arctg}\nolimits \frac{y}{L}}$, so

$$I_1 = \int\limits_0^\infty e^{-y}(y^2+L^2)^{-\frac{\alpha}{2}} e^{i(L-\alpha\mathop{\rm arctg}\nolimits \frac{y}{L})}\,idy=$$ (11.18)

$$= \int\limits_0^\infty e^{-y}(y^2+L^2)^{-\frac{\alpha}{2}} \left[... ... i\cos\left(\alpha\mathop{\rm arctg}\nolimits \frac{y}{L}-L\right) \right] \,dy$$ (11.19)

The second integral in (B.16) can be calculated by means of the substitution $x = -iy + L = \sqrt{y^2+L^2}e^{-i\mathop{\rm arctg}\nolimits \frac{y}{L}}$

$$I_2 = \int\limits_L^\infty \frac{e^{-ix}\,dx}{x^\alpha} = \int\li... ...c{\alpha}{2}} e^{i(-L+\alpha\mathop{\rm arctg}\nolimits \frac{y}{L})}\,(-i)dy =$$ (11.20)

$$= \int\limits_0^\infty e^{-y}(y^2+L^2)^{-\frac{\alpha}{2}} \left[... ... i\cos\left(\alpha\mathop{\rm arctg}\nolimits \frac{y}{L}-L\right) \right] \,dy$$ (11.21)

The imaginary parts of the integrals (B.19, B.21) cancel each other and the result is real

$$\int_L^\infty \frac{\cos kx\,dx}{x^\alpha} = k^{\alpha-1}\int\lim... ...pha}{2}} \sin\left(\alpha\mathop{\rm arctg}\nolimits \frac{y}{kL}-kL\right)\,dy$$ (11.22)