The problem of scattering on a hard sphere potential (1.48) was studied in
Sec. 1.3.2.2. In dilute systems for small interparticle distance ![]() the two
body Bijl Jastrow term
the two
body Bijl Jastrow term ![]() is well approximated by the solution
is well approximated by the solution ![]() (1.51), i.e. by the wave function of a pair of particles in vacuum. At large
distances the pair wave function asymptotically goes to a constant value, as the
particles become uncorrelated.
(1.51), i.e. by the wave function of a pair of particles in vacuum. At large
distances the pair wave function asymptotically goes to a constant value, as the
particles become uncorrelated.
Taking these facts into account we introduce the trial function in the following
way[GBC99] (here we introduce dimensionless notation by measuring the
distance ![]() in units of the hard sphere radius
in units of the hard sphere radius ![]() and energy
and energy ![]() in units of
in units of
![]() )
)
The request the function be smooth at the matching point ![]() , i.e.
, i.e.
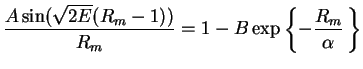 |
(2.68) |
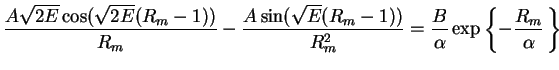 |
(2.69) |
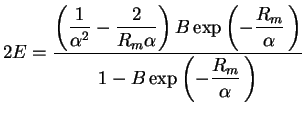 |
(2.70) |
The solution of this system is
There are three conditions for the determination of five unknown parameters, consequently two parameters are left free. The usual way to define them is minimize the variational energy in Variational Monte Carlo which yields an optimized trial wave function.